Posts filed under Just look it up (284)

David Seymour, on the Herald website

 Auckland is already denser than New York, and most American and Australian cities.  The 1.6 million people in Manhattan may live cheek-by-jowl, but not the other 20 million inhabiting the wider urban area.

An intelligent politician wouldn’t say something as apparently bizarre as this first sentence if it wasn’t true, so of course it is. The question is going to be true in what sense?

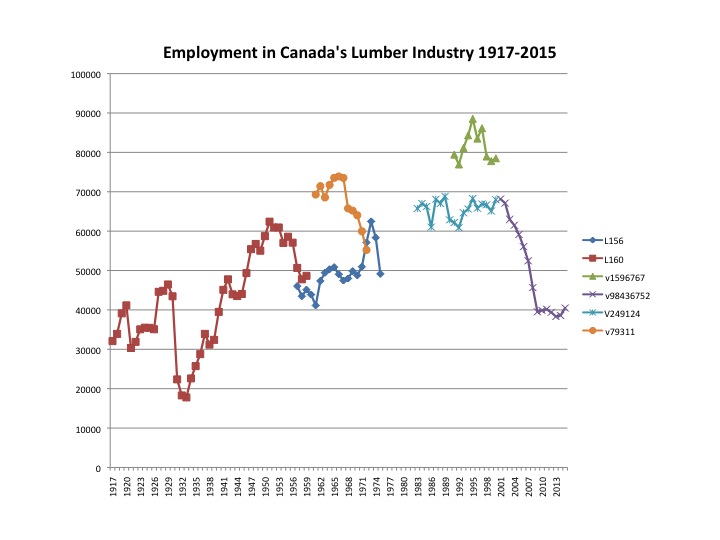
Based on the population figure, Mr Seymour is talking about the New York Metropolitan Statistical Area, aka, New York Urban Area,  which has a population of 20.1 million and a population density of 724/km²[*]. The Auckland Urban Area has a population of 1.45 million and a density of 2,600/km², and, yes, 2600 is larger than 724. However, as the scenic photos in the Wikipedia page for the New York Metropolitan Area suggest, that might not be a fair comparison.

In fact, it’s true almost by definition that the New York metropolitan area has a lower density than urban Auckland

Urban areas in the United States are defined by the U.S. Census Bureau as contiguous census block groups with a population density of at least 1,000/sq mi (390/km2) with any census block groups around this core having a density of at least 500/sq mi (190/km²).  [Wikipedia, or see full legal definition]

That is, the metropolitan area is defined as the area around New York City all the way out until the local population density is below 190/km². It’s a sensible statistical unit — the US Census Bureau wasn’t trying to make a political point about urban infill when they defined it — but it’s not the same sort of unit as Stats New Zealand’s definition of urban or metro Auckland.

So, what other comparisons could we do? We could compare the New York Metropolitan Area to the Auckland Supercity, whose population density of 320/km² is less than half as high. That might be unfair in the other direction — the Supercity is designed with the future expansion of Auckland in mind, while the US definitions are only intended for a ten-year period between censuses.

We can’t quite do the perfect comparison of redrawing Auckland Urban Area by the US rules, because NZ Area Units are bigger than US Census Block Groups, and NZ meshblocks are smaller, but someone with more time than me could try.

We could compare the Auckland urban area to genuinely urban parts of  the New York metro:  Mr Seymour mentioned Manhattan (density 27,673/km², three times that of the Auckland CBD, nine times that of the Epsom electorate) but the other four boroughs of New York City all have higher density than urban Auckland. Two of them (the Bronx, and Brooklyn) have higher density than the Auckland CBD, Queens (8237/km²) is closer in density to the Auckland CBD than to the rest of Auckland, and even Staten Island is denser than urban Auckland as a whole. In the metropolitan area but across the river from New York City proper we have Hudson County (density 5,241/km²) and Newark (density about 4500/km²). The whole of Long Island, part of the New York metropolitan area, but also known for places like Fire Island and the Hamptons, has population density 2,151/km², not far below urban Auckland.

And finally, an alternative way to do this whole comparison, which is much less sensitive to where the lines are drawn, is to look at population-weighted densities. That is, for the average person in a city, how dense is the population near them? For the whole New York metropolitan area the population-weighted density is 12000/km² (or 120/hectare). For Auckland it is 43/hectare. In other words, while people near the edges of the New York metro area have a lot of space, most New Yorkers don’t. The average person in the broad New York metropolitan area sees three times the local population density of the average Aucklander.

Update: * Mr Seymour tells me he was referring the the definition of metropolitan areas from Demographia, which trims some of the low-density parts of the Census Bureau definition of New York to give a population density of 1800, and agrees well with the StatsNZ definition of urban Auckland.  So, while the issue about the difficult in defining things comparably is still an issue, it is less his fault than I had assumed.

Family First has published an analysis that they say shows the anti-smacking law has been ineffective and harmful.  I think the arguments that it has worsened child abuse are completely unconvincing, but as far as I can tell there isn’t any good evidence that is has helped.  Part of the problem is that the main data we have are reports of (suspected) abuse, and changes in the proportion of cases reported are likely to be larger than changes in the underlying problem.

We can look at  two graphs from the full report. The first is notifications to Child, Youth and Family

The second is ‘substantiated abuse’ based on these notifications

For the first graph, the report says “There is no evidence that this can be attributed simply to increased reporting or public awareness.” For the second, it says “Is this welcome decrease because of an improving trend, or has CYF reached ‘saturation point’ i.e. they simply can’t cope with the increased level of notifications and the amount of work these notifications entail?”

Notifications have increased almost eight-fold since 2001. I find it hard to believe that this is completely real: that child abuse was rare before the turn of the century and became common in such a straight-line trend. Surely such a rapid breakdown in society would be affected to some extent by the unemployment  of the Global Financial Crisis? Surely it would leak across into better-measured types of violent crime? Is it no longer true that a lot of abusing parents were abused themselves?

Unfortunately, it works both ways. The report is quite right to say that we can’t trust the decrease in notifications;  without supporting evidence it’s not possible to disentangle real changes in child abuse from changes in reporting.

Child homicide rates are also mentioned in the report. These have remained constant, apart from the sort of year to year variation you’d expect from numbers so small. To some extent that argues against a huge societal increase in child abuse, but it also shows the law hasn’t had an impact on the most severe cases.

Family First should be commended on the inclusion of long-range trend data in the report. Graphs like the ones I’ve copied here are the right way to present these data honestly, to allow discussion. It’s a pity that the infographics on the report site don’t follow the same pattern, but infographics tend to be like that.

The law could easily have had quite a worthwhile effect on the number and severity of cases child abuse, or not. Conceivably, it could even have made things worse. We can’t tell from this sort of data.

Even if the law hasn’t “worked” in that sense, some of the supporters would see no reason to change their minds — in a form of argument that should be familiar to Family  First, they would say that some things are just wrong and the law should say so.  On the other hand, people who supported the law because they expected a big reduction in child abuse might want to think about how we could find out whether this reduction has occurred, and what to do if it hasn’t.

From the Herald, using data from Seek.co.nz: Pay shock: Wellington, not Auckland, is the New Zealand city with the highest advertised salaries.

According to the New Zealand Income Survey, the Wellington region has had the highest median weekly earnings for people in paid employment every year since at least 2007., so the shock should have had time to sink in by now. Looking at NZ.Stat, that’s also true for average weekly earnings.

However, when looking at actual earnings rather than advertisements at one site, Wellington’s percentage lead was only about half as big. And, of course, the actual dollar amounts are lower.

There’s another Herald story about incomes based on job ads at Seek.co.nz.

Data released by job search company Seek shows outside of consultancy work roles linked to the building industry were paid the most last year and were some of the few sectors to see decent pay rises.

The average salary for the construction industry is now $94,580, a boost of 5 per cent on 2013 while engineers earned an average of $92,595

Stats NZ data isn’t quite as up to date, but the NZ Income Survey, in June, found average weekly earnings in the construction industry to be $1096 (go here, and select ‘Construction’ from ‘Industry’), up about 1% from 2013 (though 5% from 2014). If you assume a full-time job with holidays, that’s $57,000 per year.

I don’t know how much of this is due to different definitions and how much is due to the Seek jobs being non-representative, but it’s possible for anyone who really cares to find out exactly what the Stats NZ number means, and that’s not true for the Seek number.

You’ve probably seen this map from Reddit: more people live inside the circle than outside it

Another map, at Stuff, claims to show the countries where “Sofia” and its variants ranks high as a name for new babies

Based on these rankings, we get

“The numbers have been crunched and the results are in. Forget John, Mohammed, Charlotte or Olivia: the most popular baby name in the world right now is Sofia.”

You can see from the map that more than half the world’s population is in countries labelled “No Data”. In fact, more than half the world’s population, plus Brazil and all of Africa. “Sofia” is the most popular baby name the way L&P is world famous in New Zealand.

But that’s not the worst bit. The first line of the story said “Forget John, Mohammed, Charlotte or Olivia”. The statistics on the map and on the linked website are for Sofia as a popular name for girls. Boys’ names aren’t in the comparison — Stuff did just ‘forget’ John and Mohammed.

You’ve got to respect Laura Wattenberg of BabyNameWizard, who does a great job getting her website into the news. Sites that take this sort of story and exaggerate it into obviously unfounded headline news, maybe not so much respect.

Official statistics agencies publish lots of useful information that gets used by researchers, by educators, by businesses, by journalists, and (with the help of groups like Figure.NZ) by everyone else.  A dilemma for these agencies is how to handle changes in the best ways to measure something. If you never change the definitions you get perfectly consistent reports of no-longer-useful information. If you do change the definitions, things don’t match up.

This graph is from a blog post by a Canadian economist, Liveo Di Matteo. It shows the number of Canadians employed in the lumber industry over time, patched together from several Statistics Canada time series.

Dr Di Matteo is a professional, and wasn’t trying to do anything subtle here — he just wanted a lecture slide — and a lot of this data was from the time when Stats Canada was among the best in the world, so it’s not a problem that’s easy to avoid. It’s just harder than it sounds to define who works in the lumber industry. For example, are the log drivers in the lumber industry, or are they something like “transport workers, not elsewhere classified”?

Update: the data Graeme Edgeler was given didn’t mean what he (reasonably) thought they meant and this analysis is no longer operative. There isn’t good evidence that the law has any substantial beneficial effect.  See Nikki Macdonald’s story at Stuff and Graeme’s own post at Public Address.

The usual objection to a “three-strikes” law imposing life sentences without parole, in addition to the objections against severe mandatory minimums, is

• It doesn’t work; or
• It doesn’t work well enough given the injustice involved; or
• There isn’t good enough evidence that it works well enough given the potential for injustice involved.

New Zealand’s version of the law is much less bad than the US versions, but there are still both real problems, and theoretical problems (robbery and aggravated burglary both include crimes of a wide range of severity).

Graeme Edgeler (who is not an enthusiast for the law) has a post at Public Address arguing that there is, at least, evidence of a reduction in subsequent offending by people who receive a first-strike warning, based a mixture of published data and OIA requests.

Here’s his data in tabular form, showing second convictions for offences that would qualify under the three-strikes law. The red cell is ‘first strike’ convictions, the other rows did not count as strikes because the law isn’t retrospective.

The first and last rows are directly comparable five-year periods. Offences that now qualify as ‘strikes’ are down 20% in the last five-year period; second convictions are down a further 62%. Data in the middle row isn’t as comparable, but there is at least no apparent support for a general reduction in reoffending in the last five-year period.

The overall 20% decrease could easily be explained as part of the long-term trends in crime, but the extra decrease in second-strike offences can’t be.  It’s also much larger than could be expected from random variation. The law isn’t keeping violent criminals off the streets, but it does seem to be deterring second offences.

Reasonable people could still oppose the three-strikes law (and Graeme does) but unless we have testable alternative explanations for the large, selective decrease, we should probably be looking at arguments that the law is wrong in principle, not that it’s ineffective.

A detail from Andrew Chen’s visualisation of all the election polls in NZ:

His full graph is somewhat interactive: you can zoom in on times, select parties, etc. What I like about this format is how clear it makes the poll-to-poll variability.  The poll result for, say, National isn’t a line, it’s a cloud of uncertainty.

The cloud of uncertainty gets narrower for minor parties (as detailed in my cheatsheet), but for the major parties you can see it span an entire 10-percentage-point grid cell or more.

This (via @albertocairo) is from the Guardian, two years ago.

At first it looks like a pie chart, but it isn’t. It’s a set of bar charts warped into a circle, so that the ratio of blue and red areas in a wedge is the square of the ratio of the numbers. Also, the circle format means the longest wedge in each pair must be the same length: 8.6% unemployment rate is the same as 4.6% military expenditure, 104% market capitalisation, and 46 Olympic gold medals.

Many of these are proportions or per-capita figures, but not all. Carbon emissions are national totals, making China look worse. Film industry revenues and exports are totals; they are also gross revenues — because the whole visual metaphor falls apart completely for numbers that can be negative. That’s why the current-year budget surplus/deficit isn’t treated like the other numbers.

There are also some unusual definitions. “Social media”, the bar where China is furthest behind, is defined just by the proportion who use Facebook, which obviously underestimates the social-media activity of the US (and also, perhaps, of China).

The post has some discussion of the difficulties — for example, the measurement and even the definition of unemployment in the two counties — and is much better than the graph.

Here’s a different take on the same countries, in the same format, from the World Economic Forum

They have similar problems with total vs proportion/mean variables. They solve the y-axis problem by working with international ranks, which at least gives a common scale. However, having 1 as the largest rank and some unspecified large number as the smallest rank does make the relationship between area and number fairly weird.  It also means that the actual numbers for each wedge aren’t fractions of a total in any sensible way.

If the main point is to be an eye-catching hook for the story, the Guardian graph is more successul

Before reading on, what proportion of New Zealand residents do you think were born overseas? (more…)