Search results for lotto (43)

There’s a big Lotto jackpot today, so it is my duty as a statistician to  write something about how people misunderstand probability. I don’t make the rules.

So, last week, I did a bogus poll on Twitter

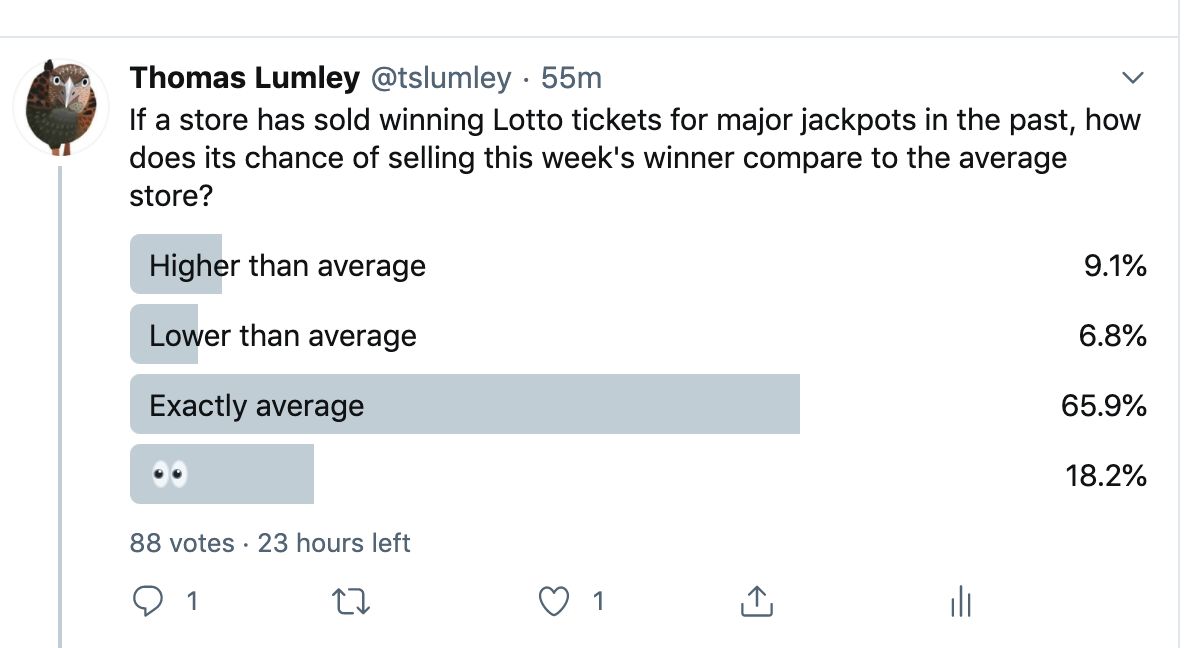
The results don’t tell you anything about any useful population,  because it was a bogus poll on Twitter, but it’s still interesting how many responses fell into the  trap.

Last week, we had headlines about ‘lucky’ stores to buy Lotto tickets.  Now, as you know, the probability that one of your chosen combinations wins does not depend at all on where you  bought the ticket, or how you chose the numbers. However, it is true that a shop which has sold winning tickets in the past is more likely to sell winning tickets in the future. Selling a winning ticket in the past is more likely for an outlet that sells lots of tickets, and an  outlet that sells lots of tickets is more  likely to sell  winning tickets in the future.  On top of that, it appears that people like to buy their tickets from outlets that have sold winning tickets in the past, which will increase the sales and therefore increase the number of future winners.  It doesn’t help the gamblers, but it does help the outlet.

In fact, saying it doesn’t help the  gamblers isn’t quite correct.   You’d hope, given the odds, that many people buying Lotto tickets were doing it primarily for entertainment (the cash return on tickets is negative, but that’s also true of beer and movies and rugby games and restaurant dinners).  Given that, anything that increases the entertainment value helps the gamblers,  and buying  from a ‘lucky’ store could count as a plus.

I also want to talk about a story in the Herald. It’s good on the  odds of winning and the impact of a ‘must win’ draw  — and quotes Dr Matt Parry, from Otago, which is generally a good indicator.  However, a separate part of the story says

Your chances of winning Powerball – one in 38 million – are less likely than you being struck by lightning – one in 280,000 – on your way to buy the ticket.

That seems not just wrong but incredibly wrong.   The story says “[m]ore than 1.9 million tickets were sold for the previous $50m must-be-won Powerball jackpot” (and that’s tickets, not lines), so at one in 280,000 we’d expect six or seven people to have been struck by lightning on their way to buy tickets.  According to this Radio NZ story, there were 13 ACC claims for lightning injury  in 3 years, and while that would leave out fatal injuries, the story also says a minority are fatal.  There’s no way you have a 1 in  280,000  chance of being struck by lightning on a routine shopping trip.

So where does this number come from? Well, the US also has a Powerball lottery, which has even lower odds of winning: one in  292 million. And there are news stories there with vaguely similar numbers.

The odds of grabbing the grand prize are 1 in 292.2 million, according to the game’s own assessment. To put this in context, your chances of being killed by a lightning strike are approximately 1 in 161,000. The odds of being killed in a shark attack are 1 in 3.7 million.

Even getting hit by a meteorite is more likely than winning the Powerball — 1 in 1.9 million.

The lightning-strike number looks to be a lifetime risk in the US, where lightning is more common than New Zealand, not the risk per shopping trip.

There are other problems with the numbers.  The 1 in 1.9 million for getting hit by a meteorite  is staggeringly wrong given that only one person in US history has been hit by a meteorite,  back in 1954.

How did the  1 in 1.9 million figure get past editing? Well, it probably wasn’t checked, but  there is a related number that’s arguably correct.  If you calculate the probability of dying due to a meteorite impact,  you have to consider the entire range of impacts from something the size of a golf  ball up to a significant asteroid.  The dinosaurs were wiped out (in part, probably) by an asteroid impact, 66 million years ago, so it would be reasonable to assume a risk in the ballpark of 1 in 100 million per year, giving a lifetime risk of experiencing the impact for an individual of one in a million or so.   Putting that together with expected  deaths from a major impact, it’s not unreasonable to get a 1 in two million risk for an individual  of dying because of an asteroid impact. On  the other hand, that’s not getting hit by a meteorite, and they shouldn’t be giving the number to two digits accuracy when even the order of magnitude must be uncertain.

So, if you’re in New Zealand and doing a careful risk assessment before buying a lottery ticket today, you probably don’t need to worry about lightning or low-flying rocks, but you should wear a mask. And if you’re in Auckland, maybe go to a local store or buy online.

Our very own Liza Bolton was summoned to TV show The Project last week to reveal how to minimise the chance of sharing a First Division Lotto win with heaps of other people.

The invite came after last Wednesday’s Lotto draw, where 40 people shared the first division prize, getting only $25,000 each rather than something with  one or two extra zeroes.

Liza had 90 seconds to share her top five tips – the first one is on the image.

Watch the clip here.

There’s a story about Lotto on Stuff that starts off promisingly

Forty Kiwis took out Lotto First Division on Wednesday night – the most first division winners in a single draw in the game’s 30-year-history.

With that many winners sharing the $1 million prize, they’re only getting $25,000 each.

This is one of the big reasons that you can’t just divide the prize by number of possible combinations and get the expected value of a ticket.

Further down, though we get this

Despite these overwhelming odds there are times when it makes mathematical sense to buy a Lotto ticket.

That’s when Powerball jackpots get so large the value of the prize pool is greater than the amount spent on tickets.

Technically, this is true. The problem is you don’t know the amount spent on the tickets, because NZ Lotto doesn’t tell anyone. So as a strategy, it’s useless.  The link goes to another story headlined Why professors of statistics play Lotto too, when the prize is big enough.  That surprised me, so I read on to see who these professors of statistics were.

There are two professors mentioned in the story, Martin Hazelton of Massey and Peter Donelan of the university currently known as Vic.  You should definitely pay attention to their opinions: Martin, in particular, is probably the country’s top statistical theorist.

They don’t, however, say they “play Lotto too, when the prize is big enough”.  Professor Hazelton doesn’t say anything on that issue. Professor Donelan is quoted right at the end of the story

“In my household, if it was up to me, I wouldn’t bother to buy one,” Donelan said.

But he suspects some stats professors do: “I expect some do regardless of what they know.”

And that’s probably true. Nothing wrong with Lotto as an entertainment — the monetary return on investment is low, but the same is true for beer, movies, rugby, or twilight walks on the beach — but it will very rarely “make mathematical sense.”

The Herald has an annoyingly uncritical story about someone who claims to have a mathematical formula for winning the lottery, rather than just being lucky.

Much more interesting: BBC’s More or Less had a story about multiple lottery wins and how they might come about.

Q: Can I improve my chances of winning Lotto by…

A: No.

Q: But….

A: No.

Q: …

A: Just no.

Q: … by buying a ticket?

A: Ok, yes. But not by very much.

Q: You sound like you’ve been asked about Lotto odds a lot.

A: There’s a larger-than-usual jackpot in the NZ Powerball

Q: Enough to make it worth buying a ticket?

A: If you like playing lotto, sure.

Q: No, as an investment.

A: I refer the honourable gentleman to the answer given some moments ago

Q: Huh?

A: No.

Q: But $35 million. And a 1 in 38 million chance of winning. And 80c tickets.  Buying all the tickets would cost less than $30 million. So, positive expected return.

A: If you were the only person playing

Q: And if I’m not?

A: Then you might have to share the prize

Q: How many other people will be playing?

A: Lotto NZ says they expect to sell more than a million tickets

Q: Compared to 38 million possibilities that doesn’t sound much

A: That’s tickets. Not lines.

Q: Ah. How many lines?

A: They don’t say.

Q: Couldn’t the media report that instead of bogus claims about a chemist in Hawkes Bay selling better tickets?

A: Probably not. I don’t think Lotto NZ tells them.

Q: That story says it would take 900 years to earn the money at minimum wage. How long to get it by playing Powerball?

A: At, say, ten lines twice per week?

Q: Sure.

A: 36900 years.

I was on Radio LIVE Drive earlier this evening, talking about lotto (way to be stereotyped). The underlying story is on Stuff

A Nelson Lotto player who won more than $100,000 playing the same numbers 12 times on the same ticket says he often picks the same numbers multiple times.

“So that when my numbers do come up, I can win a greater share of the prize.”

The player won 12 second division prizes on a single ticket bought from from Nelson’s Whitcoulls on Saturday, winning $9481 on each line, totalling $113,772.  

There’s nothing wrong with this as an inexpensive entertainment strategy. As a strategy for getting better returns from Lotto it can’t possibly work, so the question is whether it doesn’t have any effect or whether it makes the expected return worse.

In this case, it’s fairly easy to see the expected return is worse. If you play 12 lines of Lotto every week, with 12 different sets of numbers, you’ll average one week with a Division 2 win every thousand years.  If you use the same set of numbers 12 times each week, you’ll average one week with 12 Division 2 wins every twelve thousand years. You might think this factor of 12 in the odds is cancelled out by the higher winnings, but that’s only partly  true.

This week there were 25 winning Division 2 tickets, which each got an equal share of the $237,000 Division 2 prize pool. The gentleman in question held 12 of those 25 winning tickets, and so got about half the pool.  If he’d bought that set of numbers and 11 others he would have held 1 of 14 winning tickets and won, not 1/12 as much, but about 1/7th as much.   By increasing the number of winning tickets, he reduced the prize for each of his tickets, and so his strategy has slightly lower expected return than picking 12 different sets of numbers.

On the other hand, these calculations are a bit beside the point. If you play Lotto for the expected return you’re doing it wrong.

The headlines at both the Herald and Stuff say they’re about Lotto winners, but the vastly more numerous losers have to have basically the same demographics. That means any statistics drawn from a group of 12 winners are going to be very unreliable.

There some more reliable sources.  There’s (limited) information released by NZ Lotteries under the Official Information Act.  There’s also more detailed survey data from the 2012 Health and Lifestyles Survey (PDF)

Of the 12 people in today’s stories, 11 were men, even though men and women play Lotto at about the same rate. There’s a lot less variation by household income than I would have guessed. There is some variation by ethnicity, with Asians being less likely to play Lotto. People under 25 are a bit less likely to play. It’s all pretty boring.

I’ve complained a few times that clicky bogus polls have an error rate as bad as a random sample of about ten people, and are useless.  Here we have a random sample of about ten people, and it’s pretty useless.

Except as advertising.

From the Northern Advocate

An unprecedented run of success in selling winning Lotto second division winning tickets has a Whangarei store on tenterhooks expecting an even bigger win soon.

Now, in one sense this is rubbish: lotto is drawn randomly. Previous wins can’t function as an outward and visible sign of a inward propensity to sell lucky tickets, because there is no such thing.

On the other hand, statistically, you would expect a store that has sold a lot of winning tickets in the past to sell a lot of winning tickets in the future. That’s because a store that has sold a lot of winning tickets has probably just sold a lot of tickets.

A ‘lucky’ lotto vendor will usually be one that’s made a lot of profits for Lotto New Zealand. As to whether its customers are lucky, well, you don’t tend to see stories like this set in Herne Bay or Thorndon.

There are lots of Lotto strategies based on trying to find patterns in numbers.

Lotto New Zealand televises its draws, and you can find some of them on YouTube.

If you have a strategy for numerological patterns in the Lotto draws, it might be a good idea to watch a few Lotto draws and ask yourself how the machine knows to follow your pattern.

If you’re just doing it for entertainment, go in good health.

From ChCh Press

Are you feeling lucky?

The number drawn most often in Saturday night’s Lotto is one.

The second is seven, the third is lucky 13, followed by 21, 38 and 12.

And if you are selecting a Powerball for Saturday’s draw, the record suggests two is a much better pick than seven.

The numbers are from Lotto Draw Frequency data provided by Lotto NZ for the 1406 Lottery family draws held to last Wednesday.

The Big Wednesday data shows the luckiest numbers are 30, 12, 20, 31, 28 and 16. And heads is drawn more often (232) than tails (216), based on 448 draws to last week.

In theory, selecting the numbers drawn most often would result in more prizes and avoiding the numbers drawn least would result in fewer losses. The record speaks for itself.

Of course this is utter bollocks. The record is entirely consistent with the draw being completely unpredictable, as you would also expect it to be if you’ve ever watched a Lotto draw on television and seen how they work.

This story is better than the ones we used to see, because it does go on and quote people who know what they are talking about, who point out that predicting this way isn’t going to work, and then goes on to say that many people must understand this because they do just take random picks.  On the other hand, that’s the sort of journalistic balance that gets caricatured as “Opinions differ on shape of Earth.”

In world historical terms it doesn’t really matter how these lottery stories are written, but they are missing a relatively a simple opportunity to demonstrate that a paper understands the difference between fact and fancy and thinks it matters.